Inverse square y=k/x^2 graph 262697-Inverse square y=k/x^2 graph

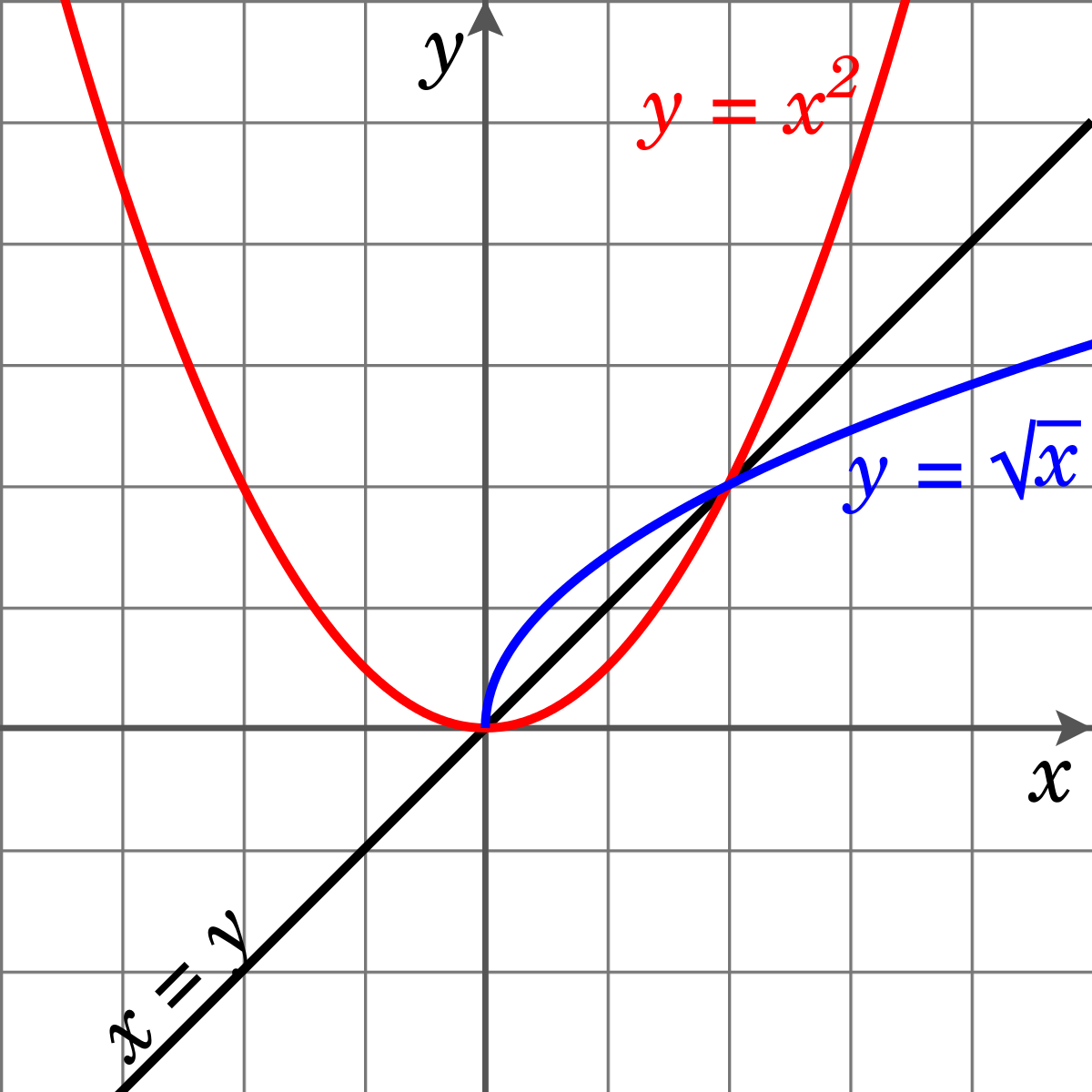
NC Math 2 Unit 6 Square Root and Inverse Variation Functions y=k·x2, where k is a negative number In this unit, you have been introduced to two new types of functions the square root function and the inverse variation function Each of these functions also has a parent graph Square root function parent graph y=√xX = k(1/y) Where "k" is a universally positive constant It can also be represented as xy = k If x and y are in inverse variation and x has two values x 1 and x 2 corresponding to y having two values y 1 and y 2 respectively, then by the definition of inverse variation, we have x 1 y 1 = x 2 y 2 = (k) In this case, it becomes x 1 / x 2Find the inverse of y = x 2 1, x > 0, and determine whether the inverse is a function You'll notice that the only difference between this and the previous example is that the domain has been restricted to the positive x axis this time

1 2

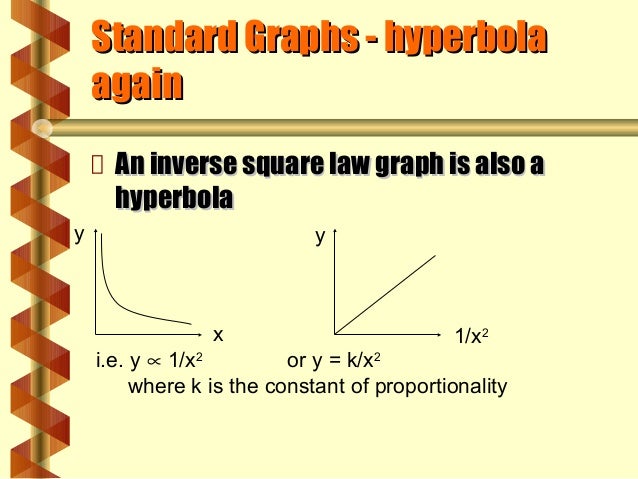
Inverse square y=k/x^2 graph

Inverse square y=k/x^2 graph-The inverse of y= 1 x2 y = 1 x 2 is x = ±√1 y x = ± 1 y The inverse function essentially maps y back to x Solution One way to easily get the inverse is to solve for the other variable InFree PreAlgebra, Algebra, Trigonometry, Calculus, Geometry, Statistics and Chemistry calculators stepbystep

Topic 1 Physics Physical Measurement

The rules from graph translations are used to sketch the derived, inverse or other related functions Complete the square to find turning points and find expression for composite functionsThe relationship is inversely related (y=k/x) because the graph is a curved line K=18x2=36, k=12x3=36, k=6x6=36 c Determine the value of constant, k Show your work K=18x2=36, k=12x3=36, k=6x6=36 The constant, k is 36 3 A typical tire pressure is 45 pounds per square inch (psi) Convert the units of pressure from psi to kilopascalsTake a function f draw its graph in the usual way;

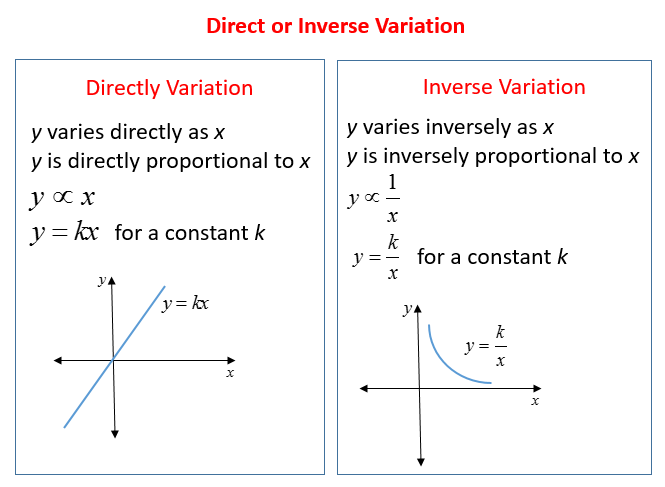
In this case, the function was a simple polynomial, so the domain was "all real numbers"The range of the original function is all the yvalues you'll pass on the graph;This y is inversely proportional to x Is the same thing as y is directly proportional to 1/x Which can be written y = k x Example 4 people can paint a fence in 3 hours How long will it take 6 people to paint it?In mathematics, an inverse function (or antifunction) is a function that "reverses" another function if the function f applied to an input x gives a result of y, then applying its inverse function g to y gives the result x, ie, g(y) = x if and only if f(x) = y The inverse function of f is also denoted as As an example, consider the realvalued function of a real variable given by f(x

The square root function is the inverse of a quadratic function with a domain limited to positive real numbers The quadratic function must be a onetoone function in order to have an inverse, so the domain is limited to one side of B Predict the effect of the parameter k on the graph of g (x) =3 Using inverse variation, what is k when y=3 and x=1 ?Free functions inverse calculator find functions inverse stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie Policy

Using Transformations To Graph Functions Of The Form

Describing Variation Graphs Station 2 The Graph Of Y Kx 2 Geogebra

Inverse of x^2 WolframAlpha Assuming "inverse" is referring to equation solving Use "inverse of" as a function16 Inverse Functions What are inverse functions?Y is inversely proportional to x y varies inversely as x y and x are inversely proportional y ∝ 1/x;

Topic 1 Physics Physical Measurement

Ch 9 10

Divide 0 0 by 4 4 Multiply − 1 1 by 0 0 Add 0 0 and 0 0 Substitute the values of a a, d d, and e e into the vertex form a ( x d) 2 e a ( x d) 2 e Set y y equal to the new right side Use the vertex form, y = a ( x − h) 2 k y = a ( x h) 2 k, to determine the values of a a, h h, and k kInterchange x and y axes, and you have the graph of the inverse function f − 1 y = f (x) means x = f − 1 (y) This can be accomplished with a drawing on a piece of paper by turning the paper over, orienting so that the old first quadrant appears in the upper right corner, and lookingSal finds the inverse of f(x)=(x2)^21 Sal finds the inverse of f(x)=(x2)^21 and then let's see we have the square root of Y minus 1 is equal to X plus 2 now we can subtract 2 from both sides we get the square root of y minus 1 minus 2 is equal to X for y is greater than or equal to 1 and so we've solved for X in terms of Y or we could

1 2

Functions And Their Graphs

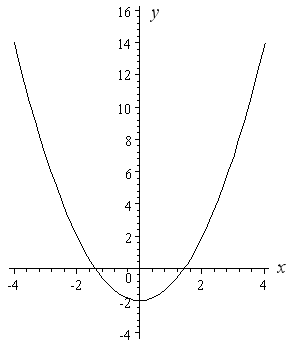
Graph the parabola, y =x^21 by finding the turning point and using a table to find values for x and yExample 2 Find the inverse function of f\left( x \right) = {x^2} 2,\,\,x \ge 0, if it existsState its domain and range This same quadratic function, as seen in Example 1, has a restriction on its domain which is x \ge 0After plotting the function in xyaxis, I can see that the graph is a parabola cut in half for all x values equal to or greater than zeroY ∝ x 2

Inversely Proportional Functions In Depth Expii

Quadratic Function Wikipedia

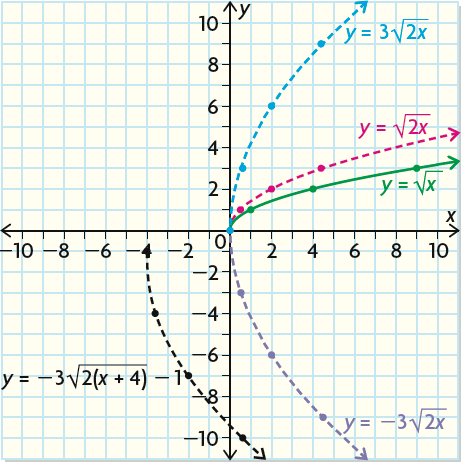
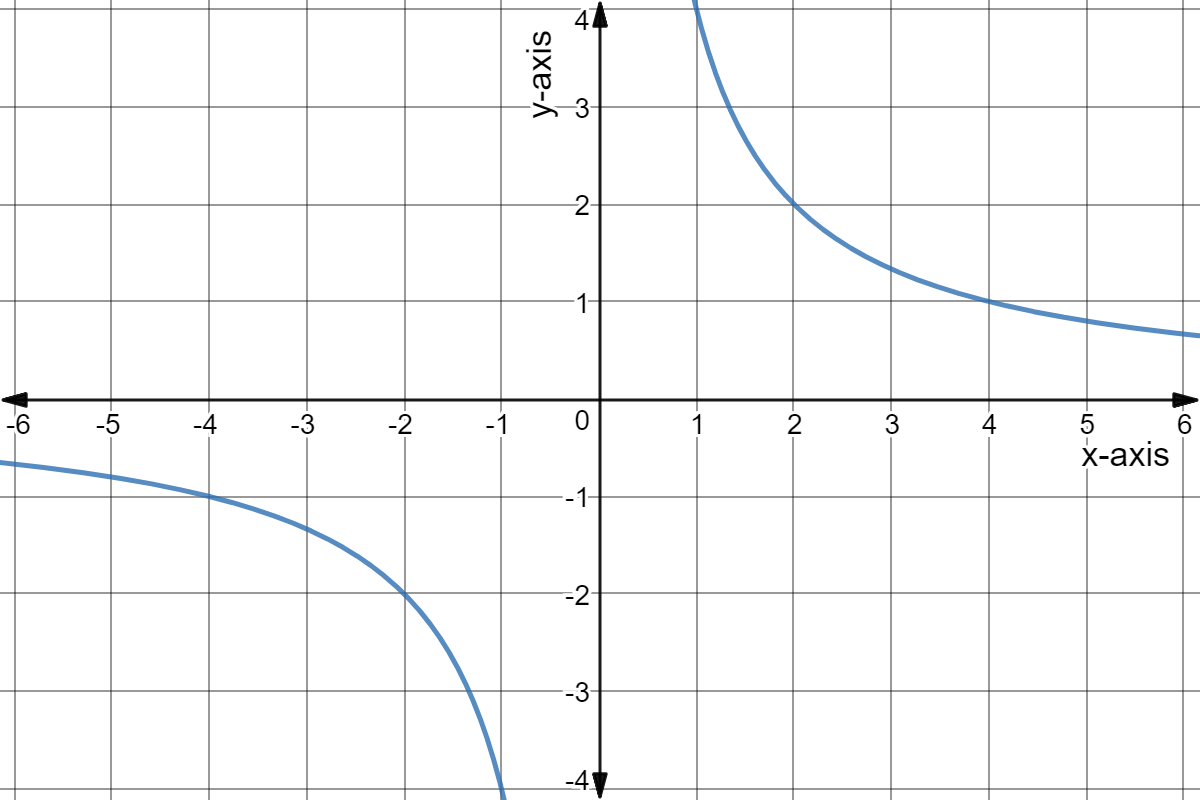
This would be of the form \(\displaystyle y=\frac{k}{{{{x}^{2}}}}\text{ or }{{x}^{2}}y=k\)) Here is a sample graph for inverse or indirect variation This is actually a type of Rational Function (function with a variable in the denominator) that we will talk about in the Rational Functions, Equations and Inequalities section hereSuppose $$ y $$ varies inversely as the square of $$ x $$ If $$ y = 5 $$ when $$ x = 3 $$, what is the value of $$ y $$ when $$ x = 1/4 $$?Substitute 4 for x and 2 for y Write the rule for inverse variation Substitute 8 for k =4(2) = 8 Step 2 Use the value of k to write an inverse variation equation y = _k x y = _8 x Step 3 Use the equation to make a table of values x421 0 124 y248 undef 842 Step 4 Plot the points and connect them with smooth curves 2 Write and graph

Mathematical Relationships Mathematical Relationships

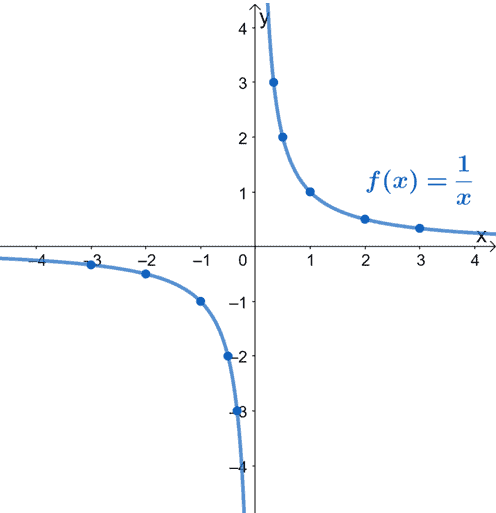
Reciprocal Function Properties Graph And Examples

 Inverse sqrtx Not a function But see below y=x^2 Since x^2 = y then x=sqrty Let f^1(x) be the inverse of y Thus, f^1(x) = sqrtx By definition, a function is a process or a relation that associates each element x in the domain of the function, to a single element y in the codomain of the functionInverse graph What type of graph?48 The following are related Directly, x=4 y=12 and then x=16, what does y=?

4 Ways To Determine Whether Two Variables Are Directly Proportional

Results From Inverse Square Law Experiment Using A Student Constructed Download Scientific Diagram

X 5x^2 is not a onetoone function, so you can't find the inverse function y = x 5x^2 y = 5(x^2 x/5) y = 5(x 01)^2 005 (x 01)^2 = (005 y)/5 xA really good resource is Inverse Functions So here we take x, add 3, and square and get an answer (call it y) So the inverse would be to take y, take the square root, and subtract 3 The graph is the same for both functions, it's just in one caseInverse relationships follow a hyperbolic pattern Below is a graph that shows the hyperbolic shape of an inverse relationship Quadratic Relationship A quadratic relationship between x and y means y is related to x^2 , x and a constant by a function, which generally represented as y = A x^2 B x C where A must be a nonzero number

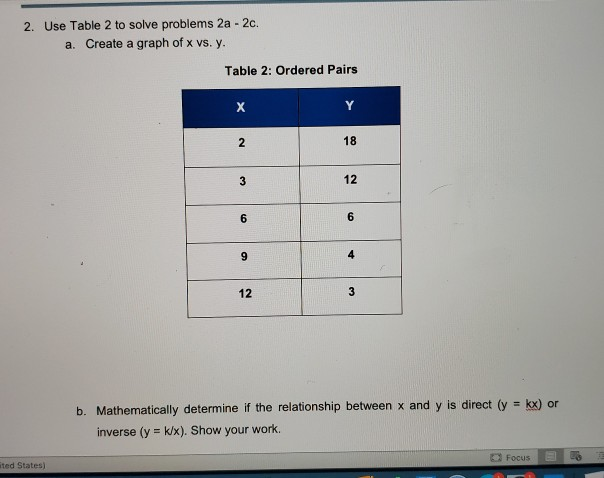
2 Use Table 2 To Solve Problems 2a 2c A Create A Chegg Com

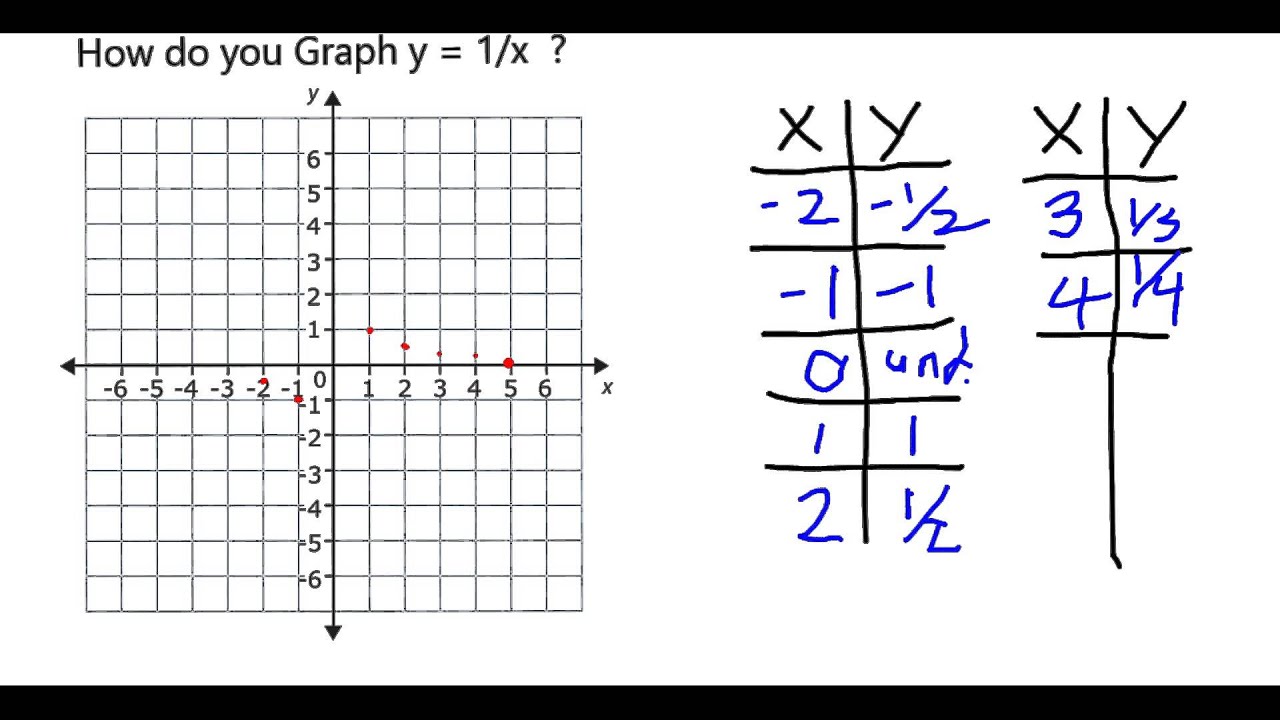
How Do You Graph Y 1 X Youtube

 Inverse Functions undo each other, like addition and subtraction or multiplication and division or a square and a square root, and help us to make mathematical "uturns" In other words, Inverses, are the tools we use to when we need to solve equations!A few coordinate pairs from the graph of the function latexy=\frac{1}{4}x/latex are (−8, −2), (0, 0), and (8, 2) If we interchange the input and output of each coordinate pair of a function, the interchanged coordinate pairs would appear on the graph of the inverse functionProduct Rule for Inverse Variation If ( x 1, y 1) and ( x 2, y 2) are solutions of an inverse variation, then x 1 y 1 = k and x 2 y 2 = k Substitute x 1 y 1 for k x 1 y 1 = x 2 y 2 or x 1 x 2 = y 2 y 1 The equation x 1 y 1 = x 2 y 2 is called the product rule for inverse variations

Http Www Phy Ilstu Edu Pte 302content Student Lab Hdbk Complex Graph Forms Pdf

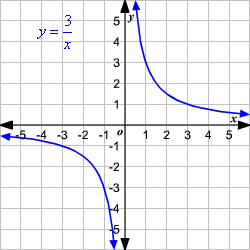
Inverse Variation

Inverse Variation Because k is positive, y decreases as x increases We can create a table that will show the comparisonExample 2 Find the inverse function, if it exists State its domain and range This function is the "bottom half" of a parabola because the square root function is negative That negative symbol is just − 1 1 −1 in disguise In solving the equation, squaring 👍 Correct answer to the question Write and graph the inverse variation in which y = 8 when x = –2 eeduanswerscom

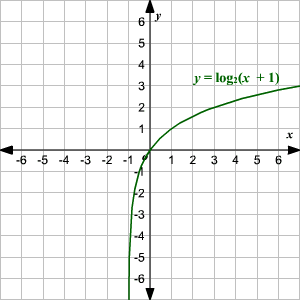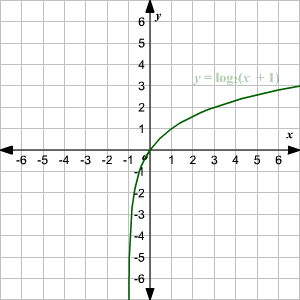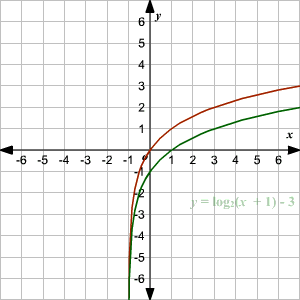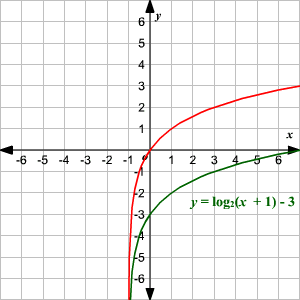
Graphing Logarithmic Functions

Pplato Flap Phys 1 3 Graphs And Measurements

Example Compare the direct variation model and the inverse variation model for when x = 2 and y = 3 Do this both numerically and graphically a Numerically Direct Variation Because k is positive, y increases as x increases So as x increases by 1, y increases by 15; Dividing the y 1equation by the y 2equation, we find That is, the ratio of yvalues is the inverse of the ratio of the squares of the corresponding values of x LIMITS As x becomes large, y becomes very small;About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us Creators

Graph Graph Equations With Step By Step Math Problem Solver

Parent Functions And Transformations She Loves Math

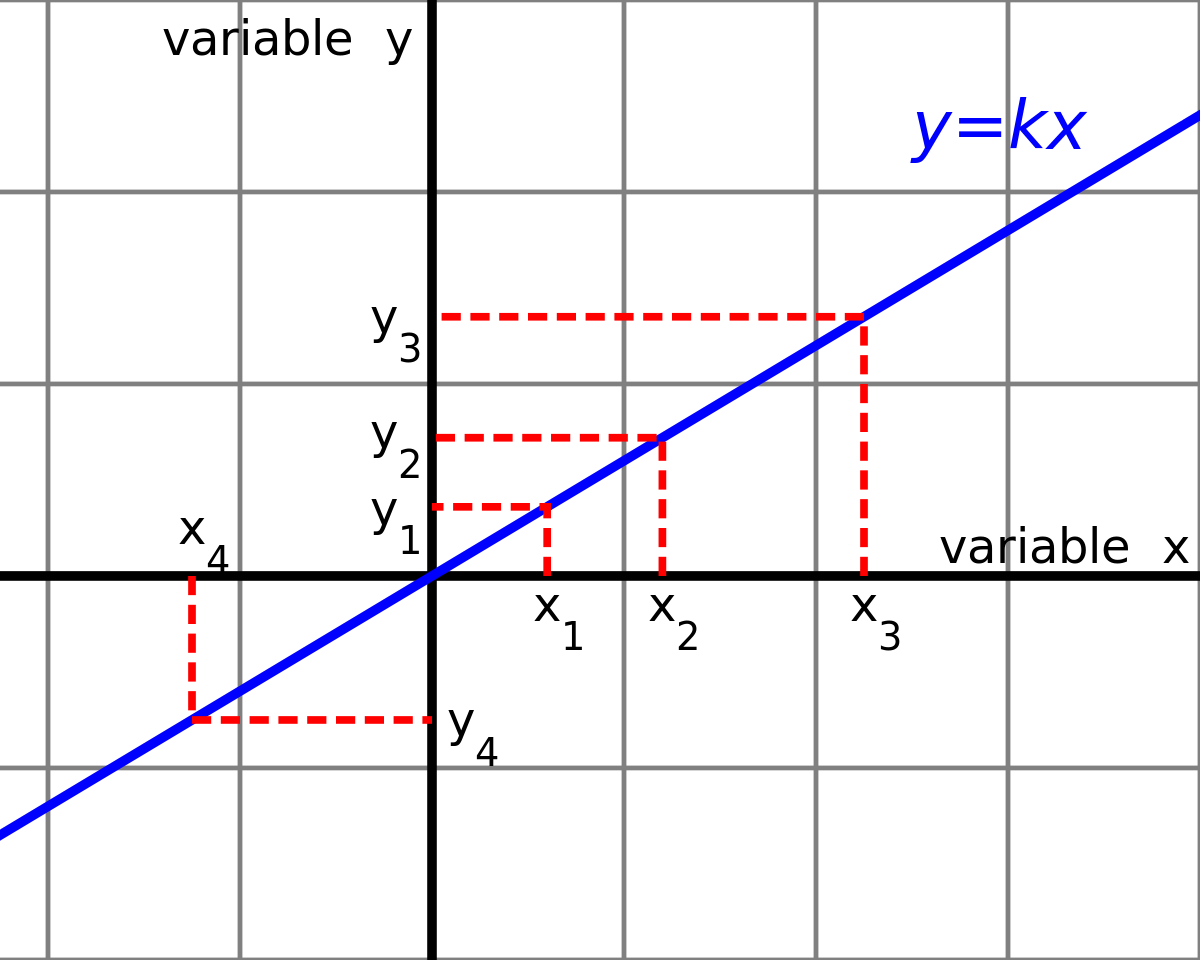
K=y/x If using Direct variation, how do you find k?3 The graph intersects y = x at kk, and kk, , where k is the constant of variation So, for this problem we have (1,1) and (1,1) as points Plot these points starting at the NEW ORIGIN Sketch the graph of 1 3 2 y x To do this 1 draw the asymptotes 2 find your vertices, but remember, this graph is in the 2nd and 4th quadrantK=xy If using Inverse variation, how do you find k?

The Characteristics Of The Graph Of A Reciprocal Function Graphs And Functions And Simultaneous Equations

2fv5d843v9w22sxtto1ibxtu Wpengine Netdna Ssl Com Wp Content Uploads 15 12 Ratio H Direct Inverse Proportion V2 Solutions Pdf

 Inverse Variation Inverse variation states that whenever the product of corresponding values of two quantities is a constant, then one quantity varies inversely as the other It is an equation that states that the product of two variables is equal to a constant In symbols, xy = k or y = k/xIn science, an inversesquare law is any scientific law stating that a specified physical quantity is inversely proportional to the square of the distance from the source of that physical quantity The fundamental cause for this can be understood as geometric dilution corresponding to pointsource radiation into threedimensional space Radar energy expands during both the signal transmission and the reflected return, so the inverse squareAs x becomes small, y becomes very large A) Consider the case in which the constant A equals 16 Plot the graph of y=16/x 2 B) Suppose the magnitude of the

State The Smallest Value Of K For Which G Has An Inverse Socratic

Graph Graph Equations With Step By Step Math Problem Solver

In this case, the straightThen the inverse is y = (x 2) / 3 If you need to find the domain and range, look at the original function and its graphThe domain of the original function is the set of all allowable xvalues;(Assume everyone works at the same rate) It is an Inverse Proportion As the number of people goes up, the painting time goes

Proportionality Mathematics Wikipedia

Direct Variation Video Lessons Examples And Solutions

An equation in which the variable is under a square rootNotation used to Represent an Inverse Function This lesson is devoted to theCalculate gradients and intercepts of the graphs and then plot them to check Solve problems involving direct and inverse

Plotting With Maple Book Chapter Iopscience

Analytic Geometry

Step 1 Use $$ \red{y = 5} $$ and $$ \blue{x = 3} $$ to find the value $$ k $$ Then write down the updated variation equationYour input find the inverse of the function $$$ y=\frac{x 7}{3 x 5} $$$ To find the inverse function, swap $$$ x $$$ and $$$ y $$$, and solve the resulting equation for $$$ x $$$ If the initial function is not onetoone, then there will be more than one inverse So, swap the variables $$$ y=\frac{x 7}{3 x 5} $$$ becomes $$$ x=\fracIf one does encounter a possible inversesquare situation, a threestep process using Graphical Analysis works beautifully to linearize the relationship When the inversesquare relationship show in the above right graph is fitted as an inverse function using a calculated column formula of 1/X, a topopening parabola results (see left graph below)

The Open Door Web Site Ib Physics Graphs

How To Draw Y 2 X 2 Interactive Mathematics

A quantity y y varies inversely with the cube of x x If y= 25 y = 25 when x =2 x = 2, find y y when x x is 6 Show Solution The general formula for inverse variation with a cube is y = k x 3 y = k x 3 The constant can be found by multiplying y y by the cube of x x k = x 3 y = 2 3 ⋅ 25 = 0 k = x 3 y = 2 3 ⋅ 25 = 0Sal finds the inverse of f(x)=(x1)^22 Sal finds the inverse of f(x)=(x1)^22 1 to both sides you get negative square root of y plus 2 plus 1 is equal to X for 4 y is greater than or equal to negative 2 or if we want to rewrite it we could say that X is equal to the negative square root of y plus 2 plus 1 for y is greater than equal toY = a√ (xh) k Square Root Graphing Radical Equations *Since positive AND negative numbers can be cube rooted, graph has 2 bends Graphing Radical Equations Example y = a∛ (xh) k (h, k) = (0, 0) a = 1 right 1 & up 1 a = 1 left 1 & down 1

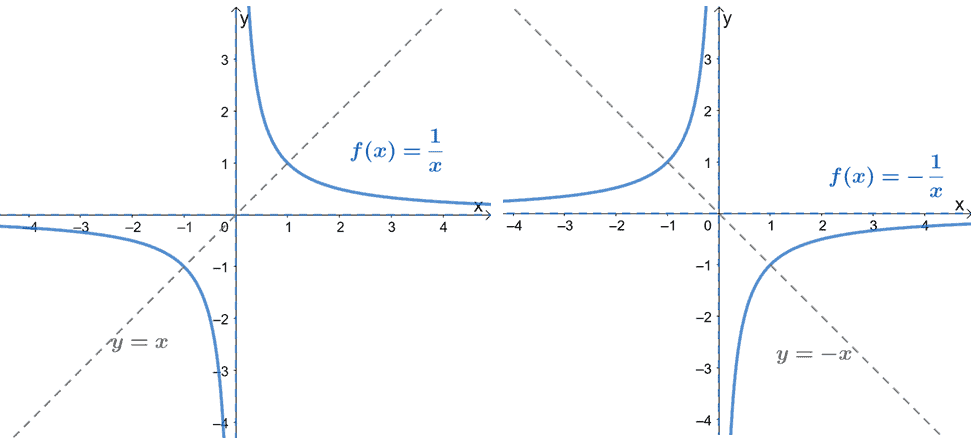
Reciprocal Function Properties Graph And Examples

Graphing A Translation Of A Rational Function Study Com

3x 2y = 1 Plot families of exponential and reciprocal graphs For example y = 2 x, y = 3 x, y = 4 x y = 1÷x, y = 2÷x, y = 3÷x, Reduce a given linear equation in two variables to the standard form y = mx c;Y ∝ x 1 If n = 2, ie y ∝1/x 2, we call this an inverse square We can describe an inverse square relationship using the following language y is inversely proportional to the square of x y varies inversely as x 2 y ∝ 1/x 2; It is technically find to write the inverse function as #x=f^{1}(y)=y^22# However, for the purposes of graphing both functions in the same picture and seeing the reflection property across the line #y=x#, you can now swap the variables and write #y=f^{1}(x)=x^22# (If the variables represent specific reallife quantities, you should not do this swapping)

Inverse And Joint Variation College Algebra

What Is The Difference Between Direct Proportional Proportional Non Proportional And Inversely Proportional Graphs Quora

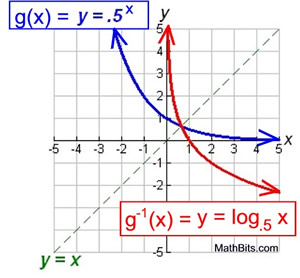
Exponential Functions Mathbitsnotebook Ccss Math

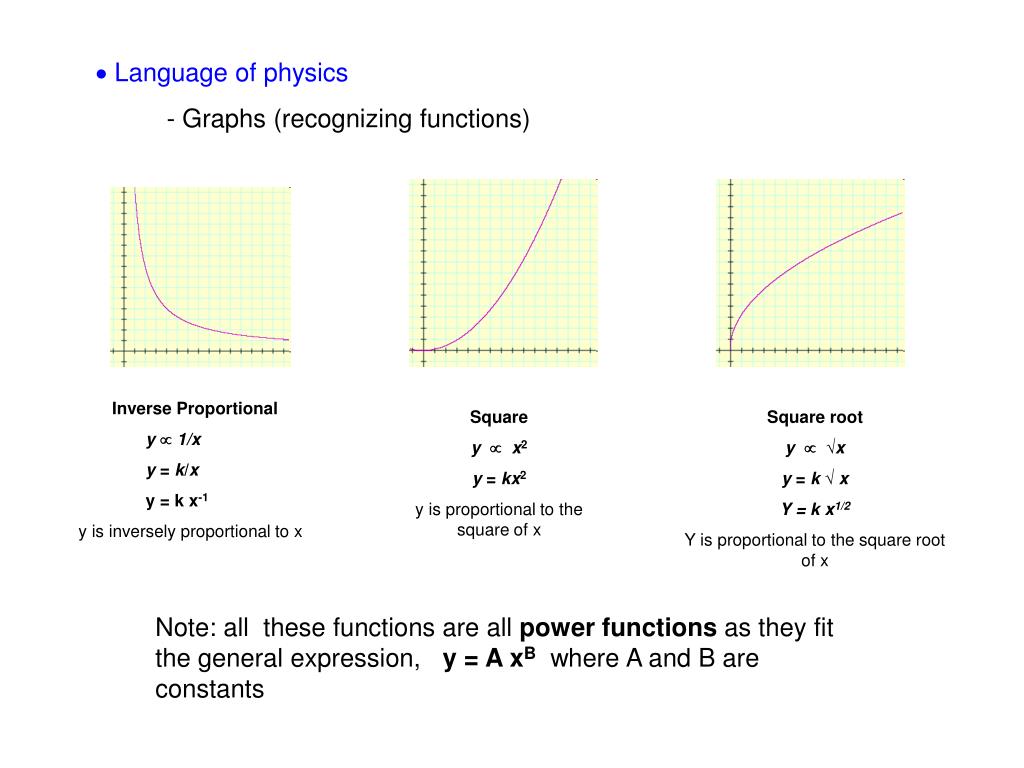
Ppt What Is Physics Powerpoint Presentation Free Download Id

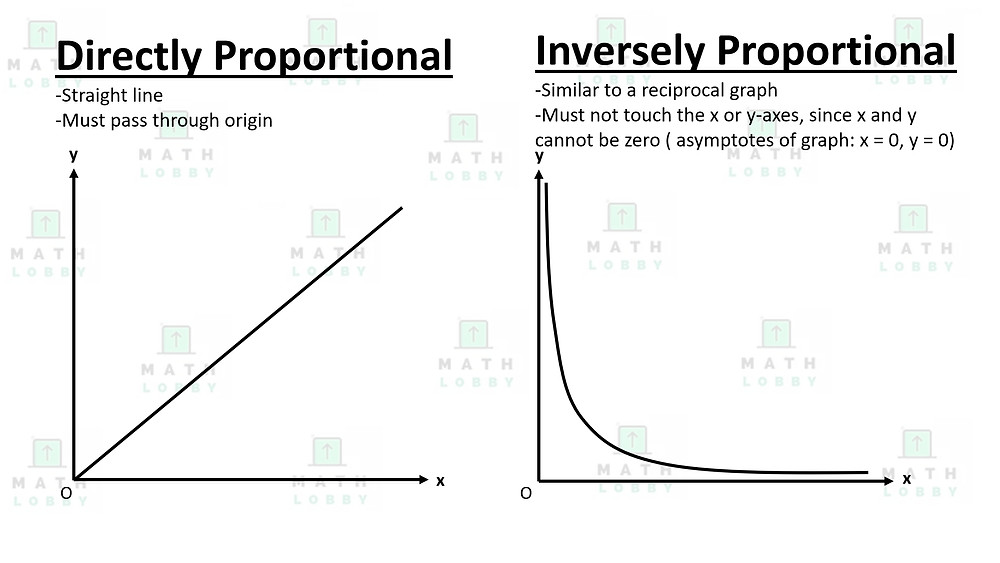
Direct And Inverse Proportion

Direct Variation Free Math Help

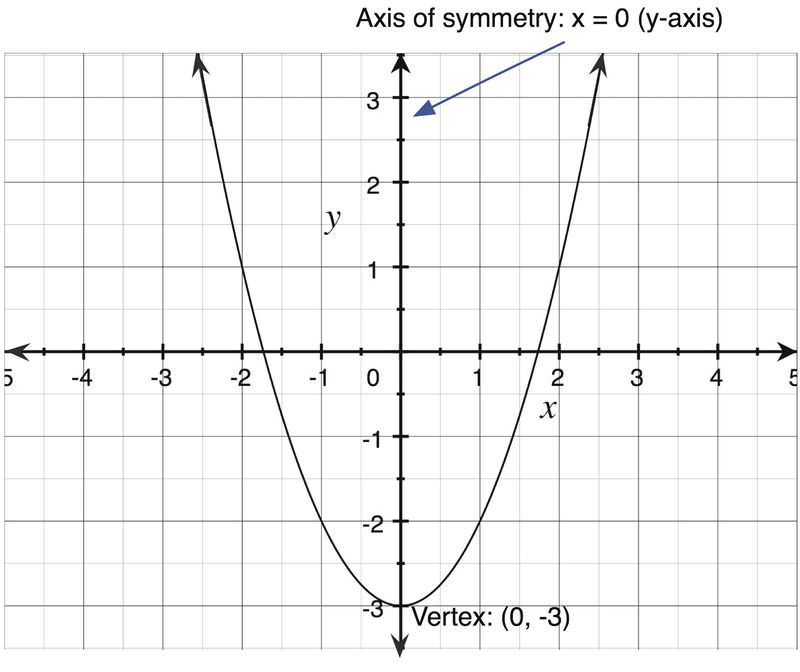
Square And Cube Function Families Read Algebra Ck 12 Foundation

Direct Inverse Variation

The Characteristics Of The Graph Of A Reciprocal Function Graphs And Functions And Simultaneous Equations

Linearize An Exponential Looking Graph With Log Function Mathematics Stack Exchange

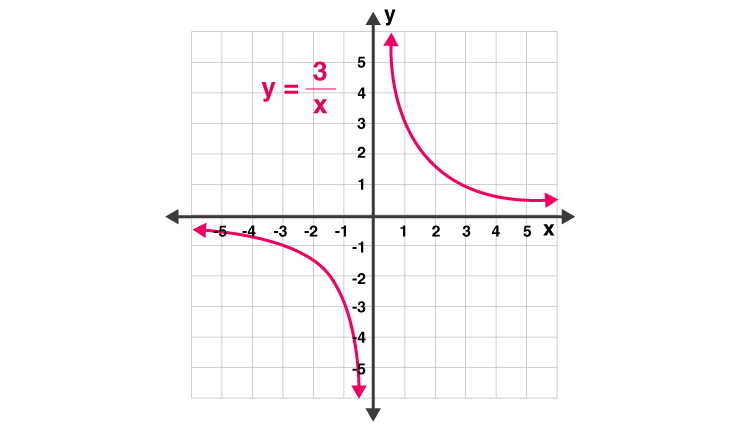
Inverse Variation Definition Formula Equation Questions

Function Definition Types Examples Facts Britannica

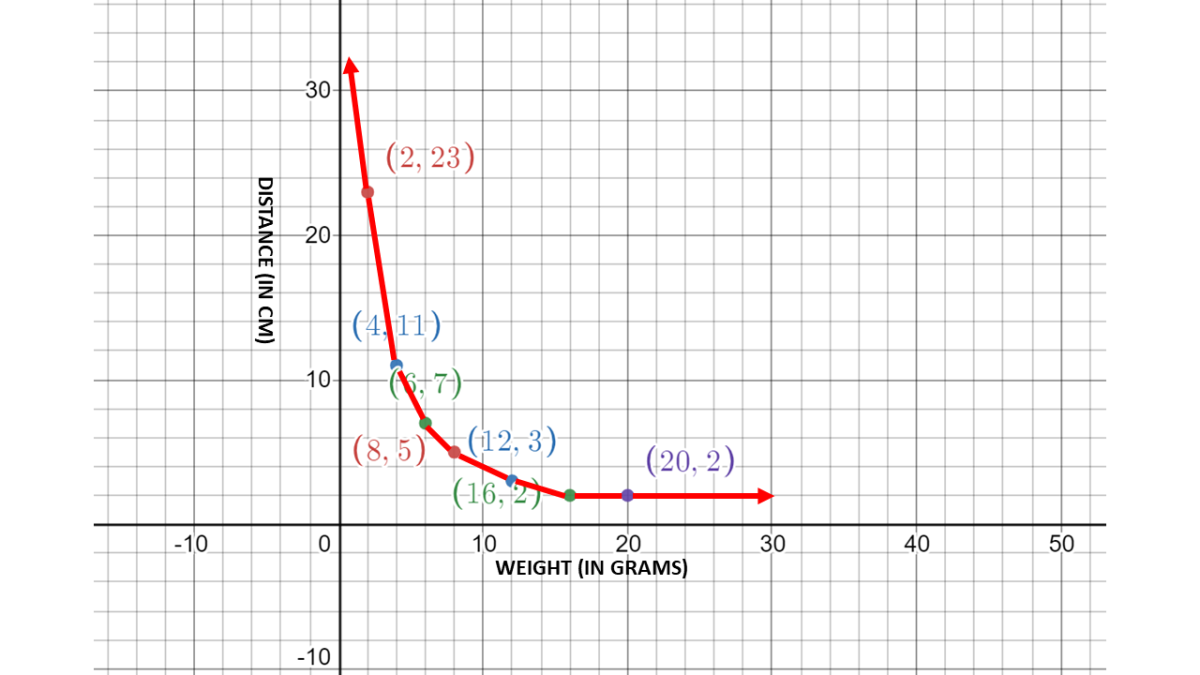
Inverse Variation Definition Formula Graph And Examples Owlcation

Http Www Mpsaz Org Rmhs Staff Lxcoleman Trig Test Practice Files Review Chapter 3 Notes Solutions Pdf

1

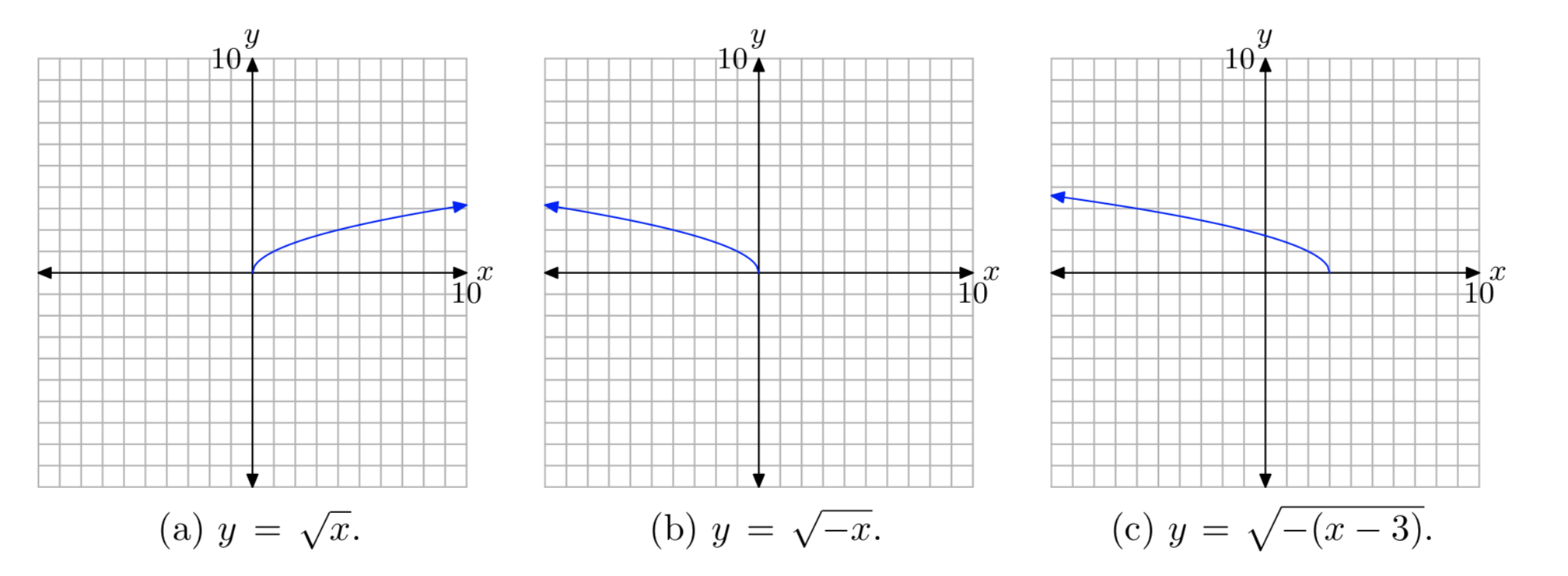
9 1 The Square Root Function Mathematics Libretexts

Test Algebra Nation Section 4 Vocabulary Linear Equations Functions And Inequalities Quizlet

Www Brainfuse Com Jsp Alc Resource Jsp S Gre C Cc 10

Inverse Relationships

Variation College Algebra

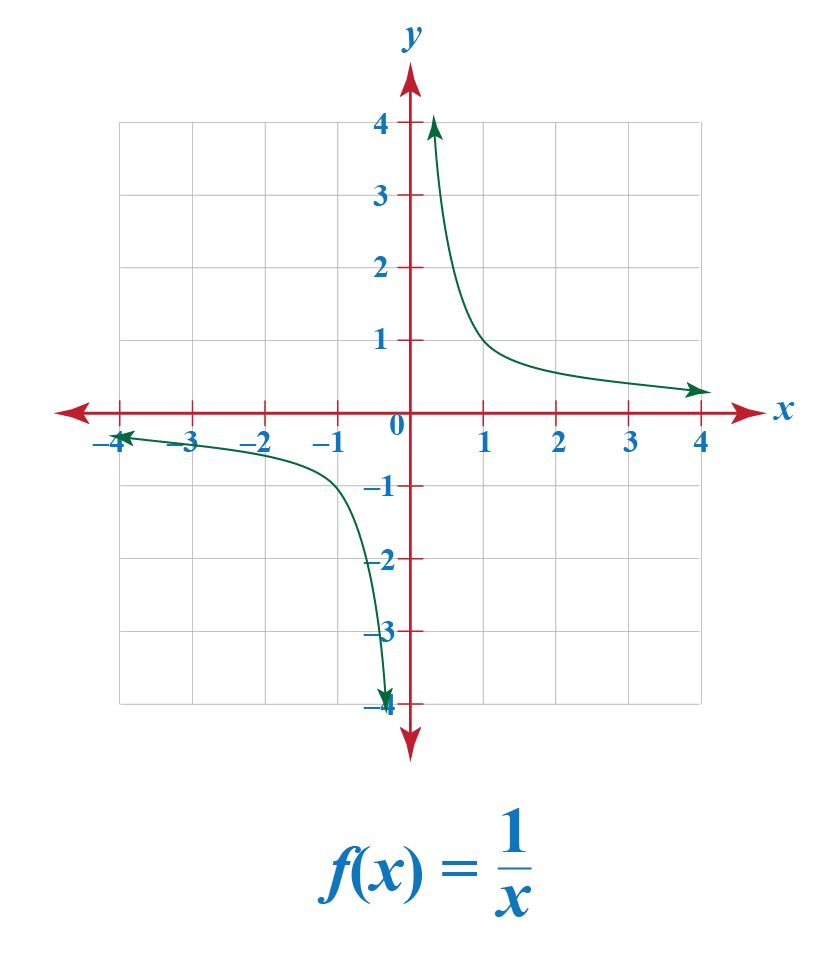
Reciprocal Functions Graphs Calculator Examples Cuemath

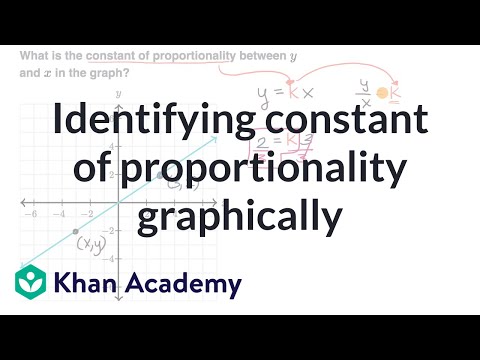
Identifying Constant Of Proportionality Graphically Video Khan Academy

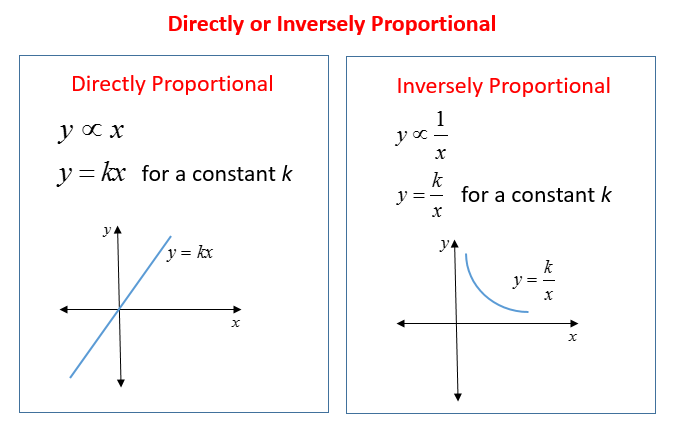
Proportion Word Problems Videos Lessons Examples

Graphs Of Number Relationships

Direct Variation Equation Graph Formula And Examples Owlcation

Inverse Of Constant Function Chilimath

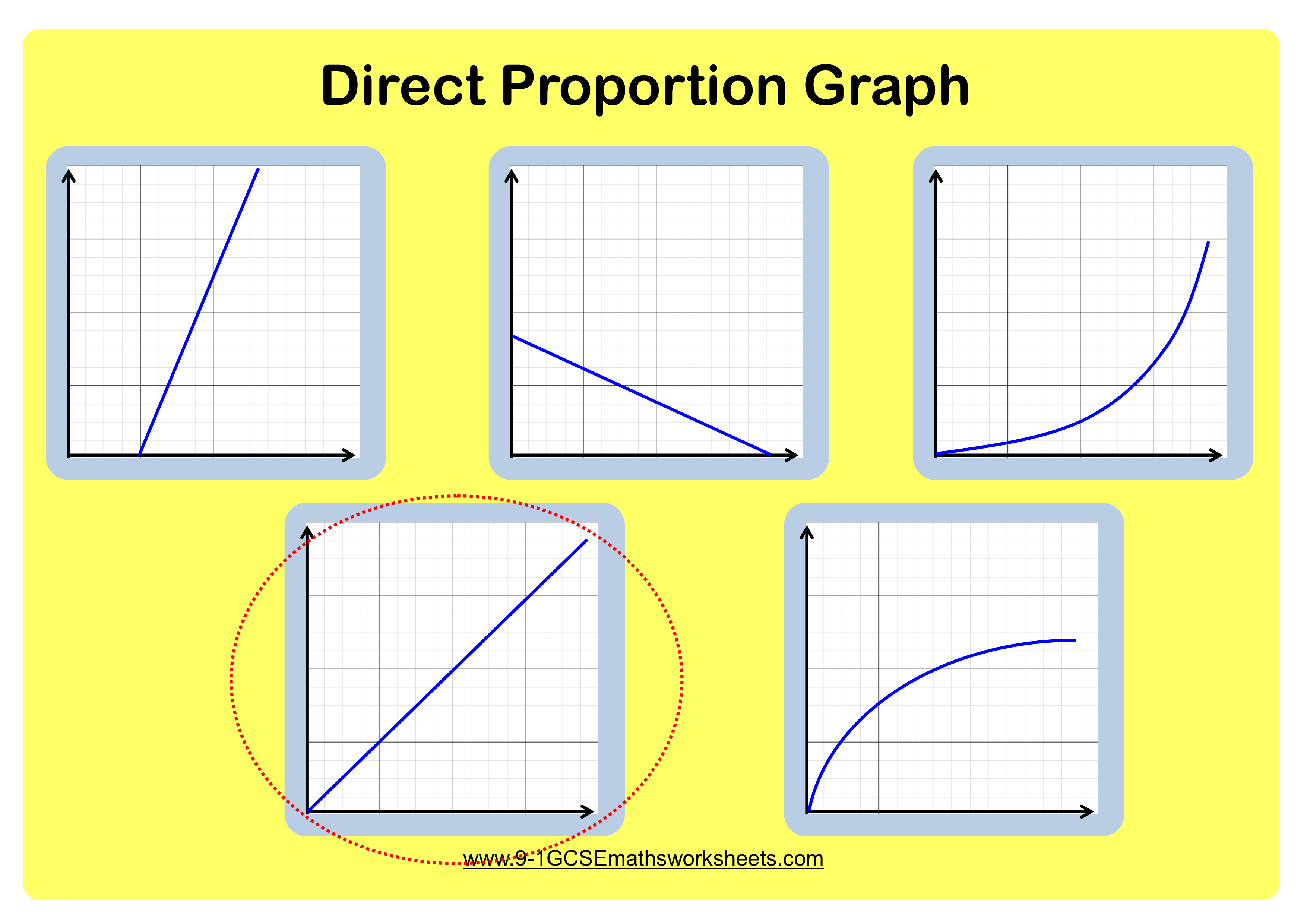
Direct Proportion Worksheets New Engaging Cazoomy

Reciprocal Function Explanation Construction Of Graph And Solved Examples

What Is The Graph Of Y X Quora

1

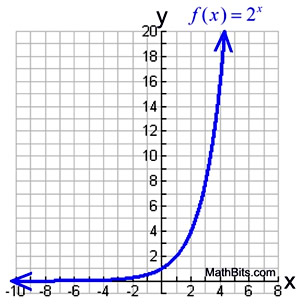
Exponential Functions Mathbitsnotebook Ccss Math

1

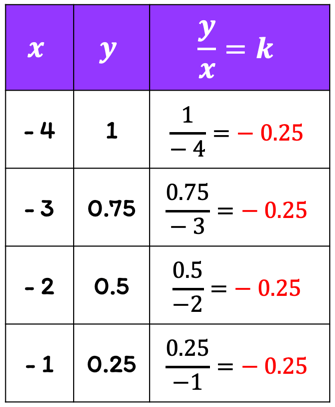
Direct Variation Chilimath

Proportionality Mathematics Wikipedia

2

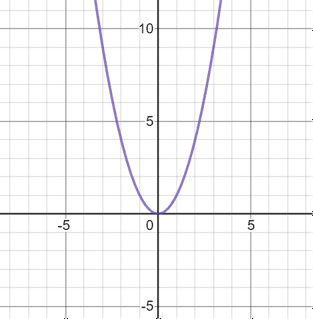
Parabola

Rational Functions Algebra And Trigonometry

Reciprocal Functions Definition Examples Graphs Video Lesson Transcript Study Com

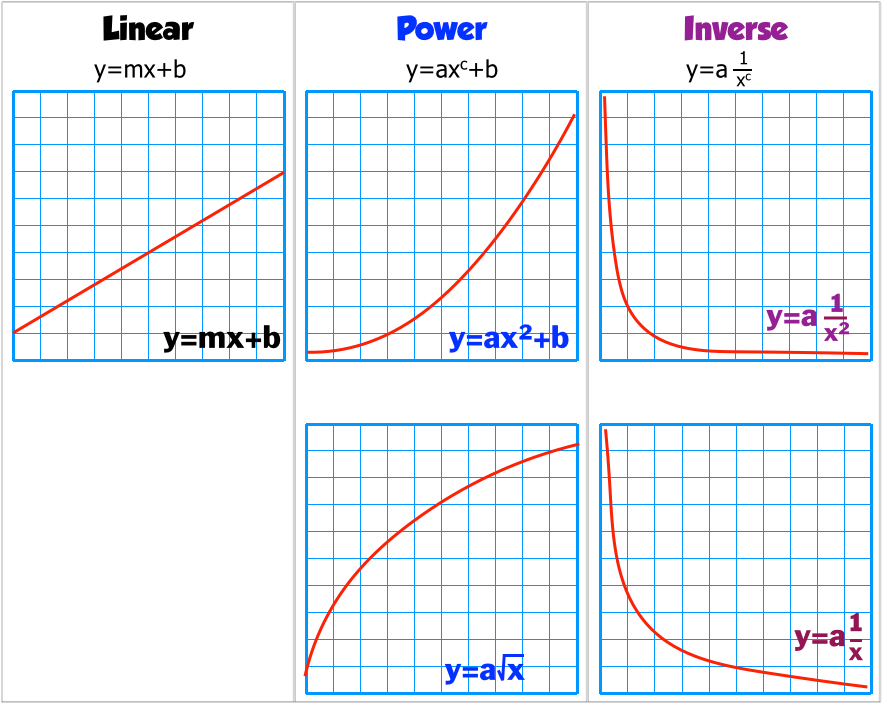
Linearizing Data

All Elementary Mathematics Study Guide Functions And Graphs Elementary Functions And Their Graphs

Rational Expressions And Their Operations Flashcards Quizlet

Using Linear Quadratic Inverse Inverse Square Graphs To Understand Proportionality Ib Physics Youtube

The Science Of Physics Chapter 1 Holt 1 1 What Is Physics Physics Is The Scientific Study Of Matter And Energy And How They Interact With Each Other Matter Ppt Download

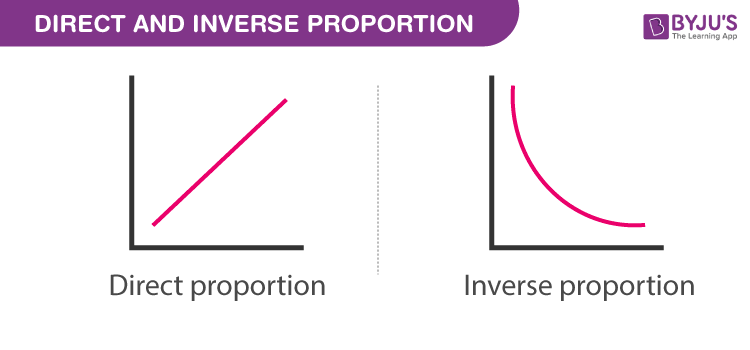
Inversely Proportional Definition Formula And Examples

Scaling And Translating Quadratic Functions

Integrated Rate Laws

Exponetials And Growth Functions

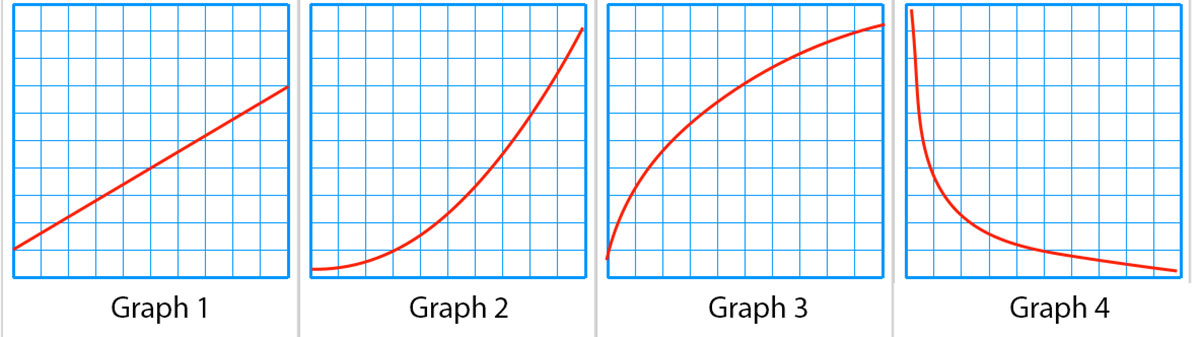
Mathematical Relationships Mathematical Relationships

Inverse Square An Overview Sciencedirect Topics

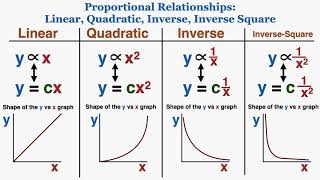
Using Linear Quadratic Inverse Inverse Square Graphs To Understand Proportionality Ib Physics Youtube

2fv5d843v9w22sxtto1ibxtu Wpengine Netdna Ssl Com Wp Content Uploads 15 12 Ratio H Direct Inverse Proportion V2 Solutions Pdf

File Inverse Square Graph Svg Wikimedia Commons

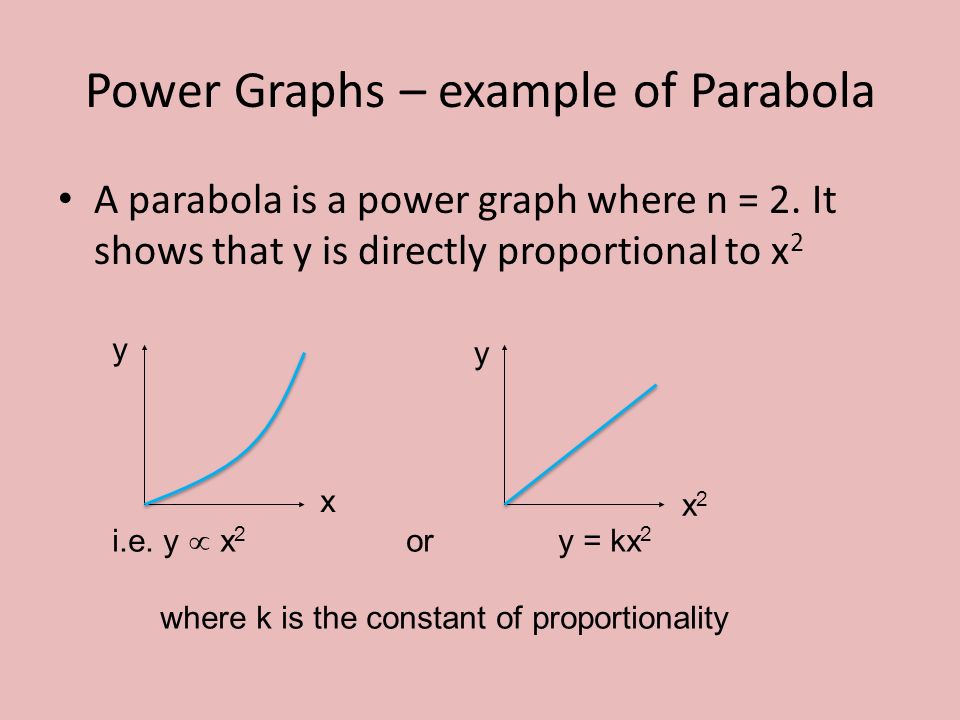
Proportional Techniques Standard Graphs Proportional Techniques Ppt Download

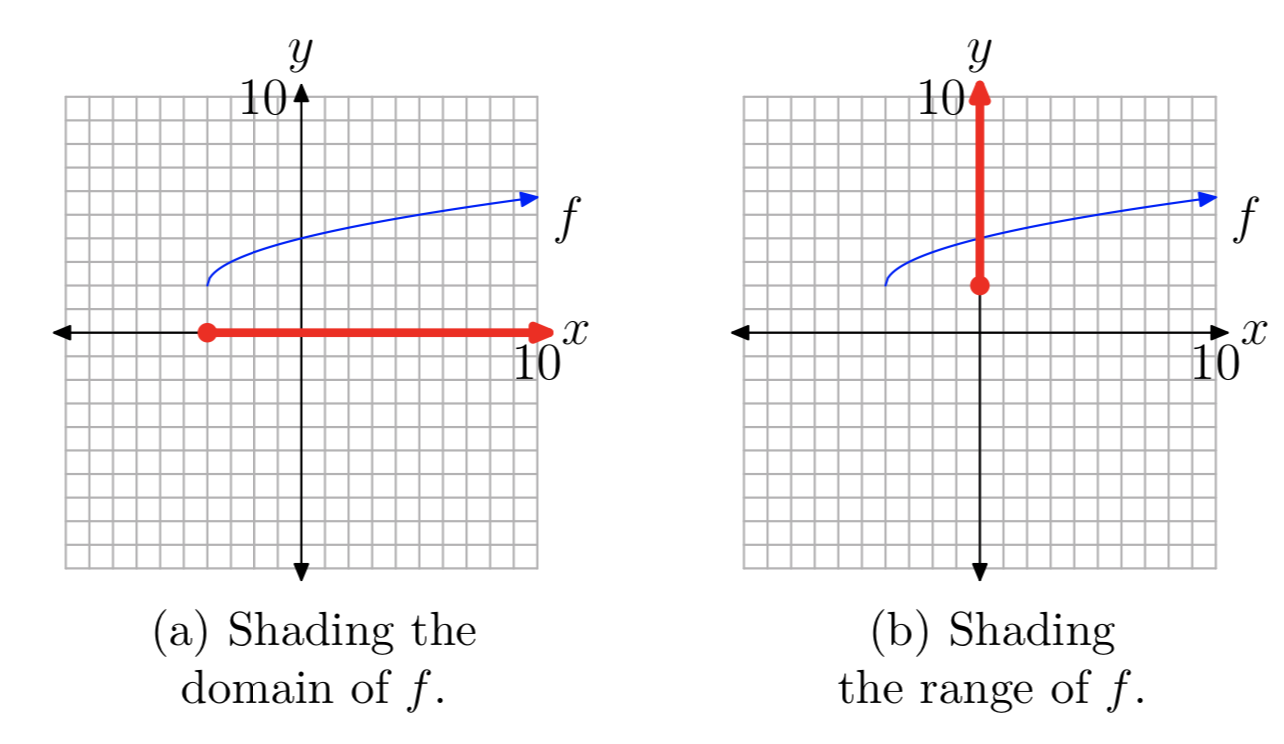
9 1 The Square Root Function Mathematics Libretexts

Direct And Inversely Proportional Quantities Concepts Videos Examples

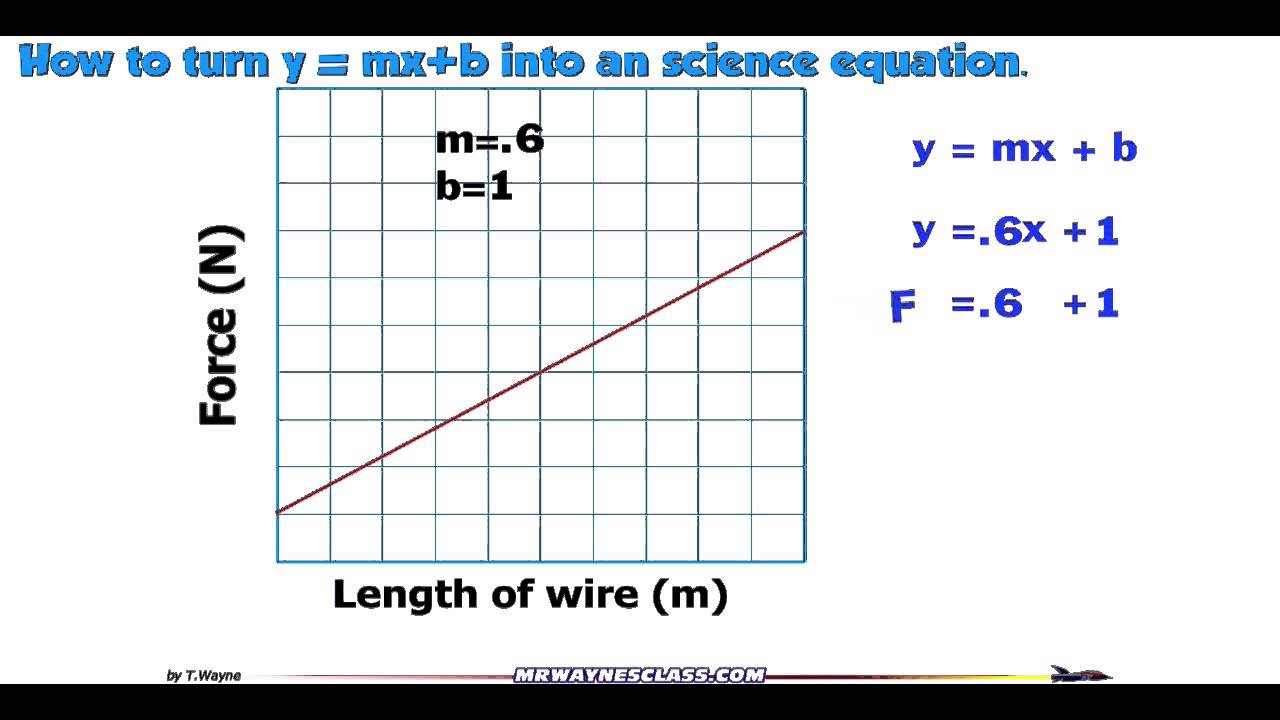
Linearizing Data

Solution In A Quadratic Variation Graph What Does It Look Like When Y Equals 18 When X Equals 6 What Does The Rest Of The Graph Look Like

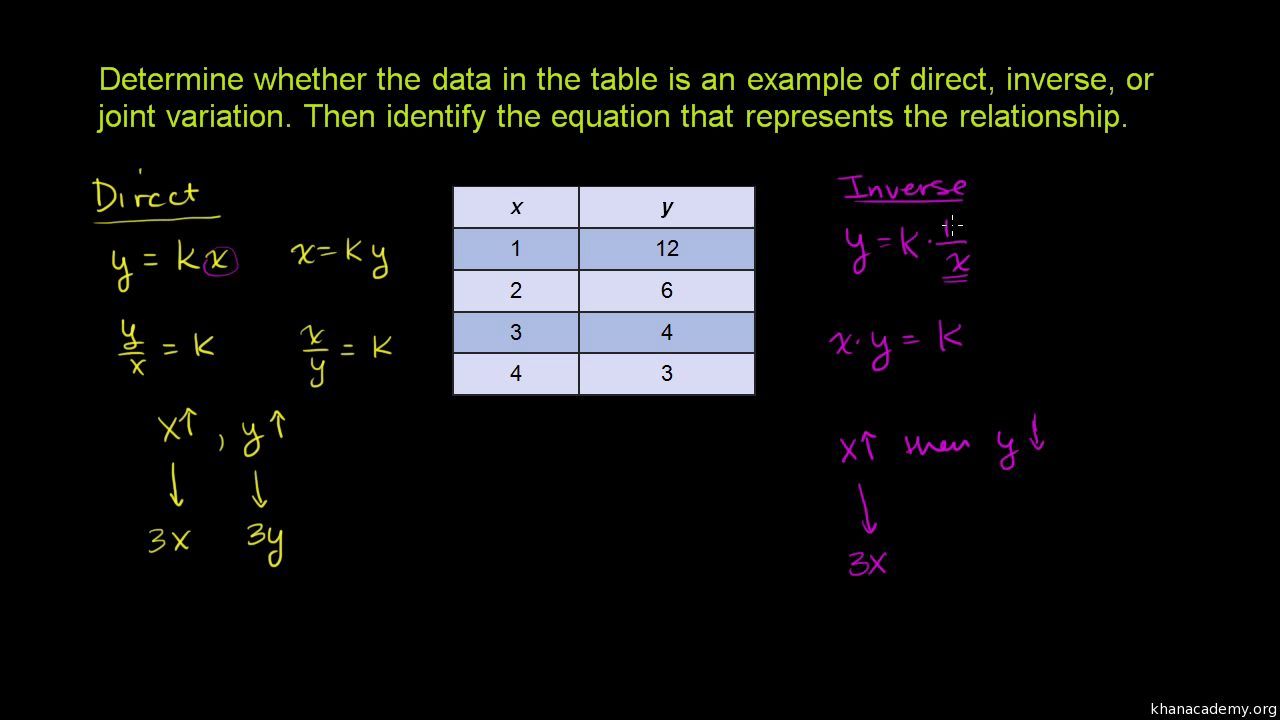
Recognizing Direct Inverse Variation Table Video Khan Academy

Inverse Relationships

Times Module M35 Proportion

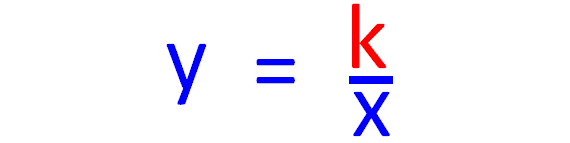
Constant Of Proportionality

Pendulum Mock Lab Ppt Video Online Download

2 Use Table 2 To Solve Problems 2a 2c A Create A Chegg Com

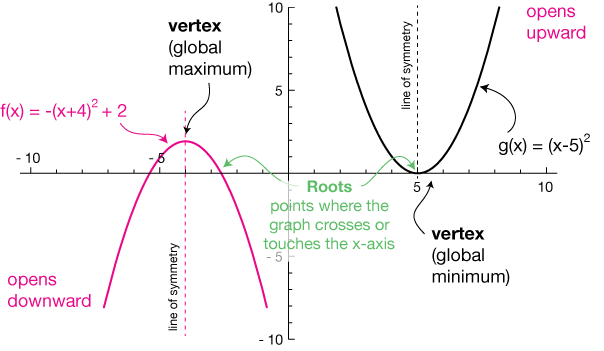
Quadratic Functions

Times Module M35 Proportion

File Inverse Square Graph Svg Wikimedia Commons

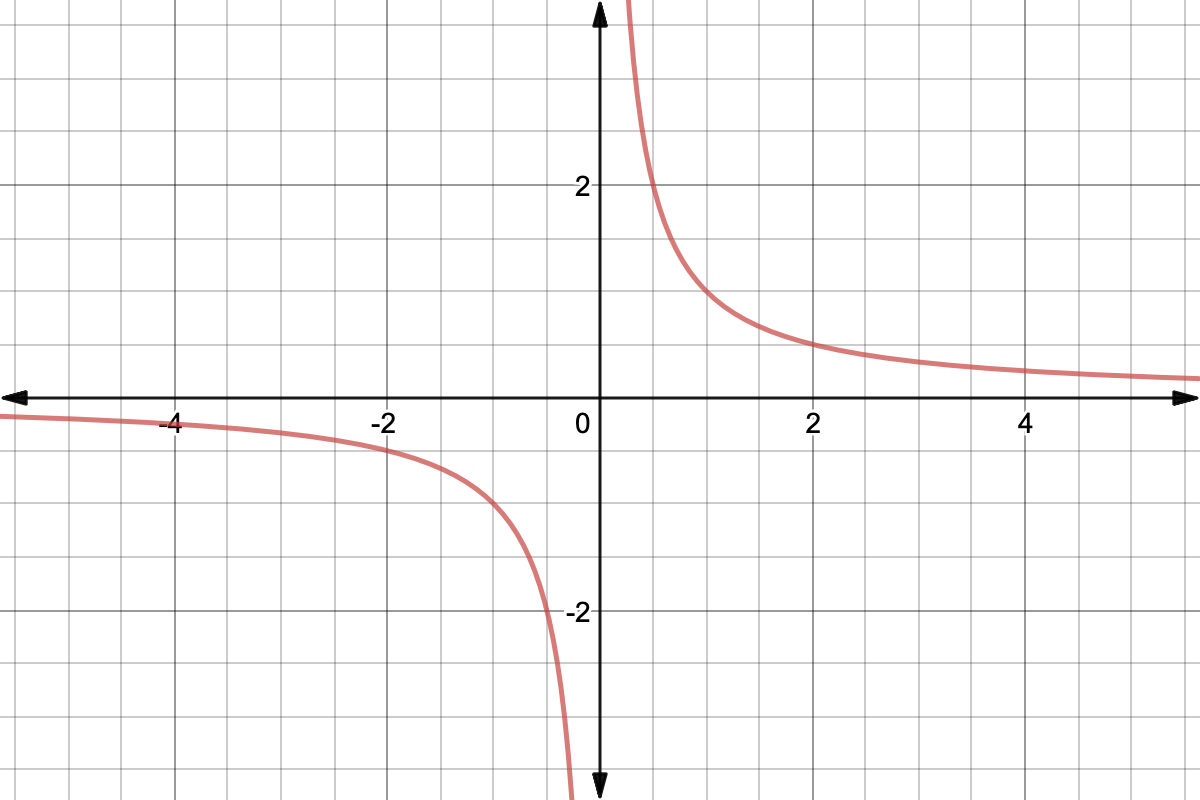
Inversely Proportional Functions In Depth Expii

The Open Door Web Site Ib Physics Graphs

Explore Y K X Inverse Variation

Group Activity Graphing Direct And Inverse Proportions

1

Chapter 3 1 Variation Ppt Video Online Download

Incoming Term: inverse square y=k/x^2 graph,